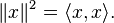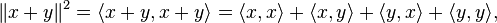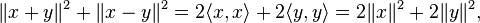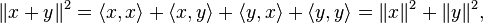parallelogram

The parallelogram law in inner product spaces

Vectors involved in the parallelogram law.

In a normed space, the statement of the parallelogram law is an equation relating norms:

2|x|^2+2|y|^2=|x+y|^2+|x-y|^2. \,

In an inner product space, the norm is determined using the inner product:

|x|^2=langle x, x angle.\,

As a consequence of this definition, in an inner product space the parallelogram law is an algebraic identity, readily established using the properties of the inner product:

|x+y|^2=langle x+y, x+y angle= langle x, x angle + langle x, y angle +langle y, x angle +langle y, y angle, \,

|x-y|^2 =langle x-y, x-y angle= langle x, x angle - langle x, y angle -langle y, x angle +langle y, y angle. \,

Adding these two expressions:

|x+y|^2+|x-y|^2 = 2langle x, x angle + 2langle y, y angle = 2|x|^2+2|y|^2, \,

as required.

If x is orthogonal to y, then 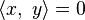$langle x , y angle = 0$ and the above equation for the norm of a sum becomes:

|x+y|^2= langle x, x angle + langle x, y angle +langle y, x angle +langle y, y angle =|x|^2+|y|^2,

which is Pythagoras' theorem.